Help ?

IGMIN: We're glad you're here. Please click 'create a new query' if you are a new visitor to our website and need further information from us.

If you are already a member of our network and need to keep track of any developments regarding a question you have already submitted, click 'take me to my Query.'

Genomics Anxiety Soil Science Rhinology Immunology Information Technology Physical Therapy Molecules Oncology Nutritional Disorders Emergency Medicine Biotechnology Otolaryngology Physiology Evolutionary Biology Dermatology Data Engineering Metabolism Ophthalmology Pharmaceutica Analytica Acta
    

Focusing on Biology, Medicine and Engineering ISSN: 2995-8067 | Quick Google Scholar

Search

Organised by  IgMin Fevicon

Languages

Submit Manuscript

Browse by Subjects

Welcome to IgMin Research – an Open Access journal uniting Biology, Medicine, and Engineering. We’re dedicated to advancing global knowledge and fostering collaboration across scientific fields.

Biology
Medicine
Engineering
General Science
A to Z Topics

Members

We focus on accelerating knowledge through shared interdisciplinary collaboration.

Editors
Authors
Join Now as Editor

Articles

We focus on accelerating knowledge through shared interdisciplinary collaboration.

Early Online
Current Issue
Previous Issue
Archive
Browse by Universities
Table of Content

Explore Content

We focus on accelerating knowledge through shared interdisciplinary collaboration.

Identify Us

Publish Now

Policies

Manuscript Guidelines

Other Services

Identify Us

We focus on accelerating knowledge through shared interdisciplinary collaboration.

About Us
Contact us
Testimonials
IgMin Corporation

Welcome to IgMin, a leading platform dedicated to enhancing knowledge dissemination and professional growth across multiple fields of science, technology, and the humanities. We believe in the power of open access, collaboration, and innovation. Our goal is to provide individuals and organizations with the tools they need to succeed in the global knowledge economy.

Publications Support
publications.support@igmin.org
E-Books Support
ebooks.support@igmin.org
Webinars & Conferences Support
webinarsandconference@igmin.org
Content Writing Support
contentwriting.support@igmin.org
IT Support
it.support@igmin.org
IgMin Youtube Link

Search

Select Language

Explore Section

Content for the explore section slider goes here.

179 of 183
Multicenter Molecular Integrals over Dirac Wave Functions for Several Fundamental Properties
Kazuhiro Ishida
181 of 183
Preparing for SpaceX Mission to Mars
Donald Rapp
Abstract

Abstract at IgMin Research

We focus on accelerating knowledge through shared interdisciplinary collaboration.

General-science Group Review Article Article ID: igmin289

General Solutions for MHD Motions of Viscous Fluids with Viscosity Linearly Dependent on Pressure in a Planar Channel

Physics DOI10.61927/igmin289
Constantin Fetecau * 1 and
Costică Moroşanu 2
Affiliation

Affiliation

    1Academy of Romanian Scientists, 3 Ilfov, Bucharest 050044, Romania

    2Department of Mathematics, “Alexandru Ioan Cuza” University, Iasi 700506, Romania

Fulltext HTML Fulltext PDF Cite this article
0
REFERENCES
1.6k
VIEWS
167
DOWNLOADS
145
Similar Articles
Connect with Us

Abstract

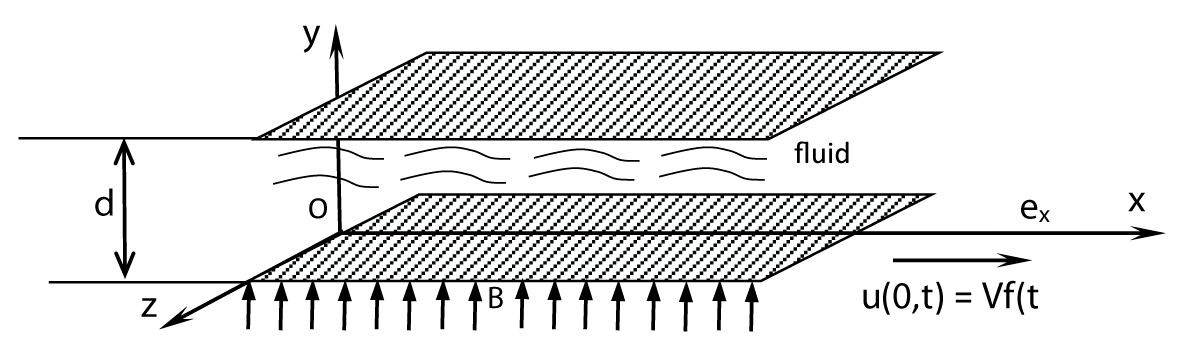
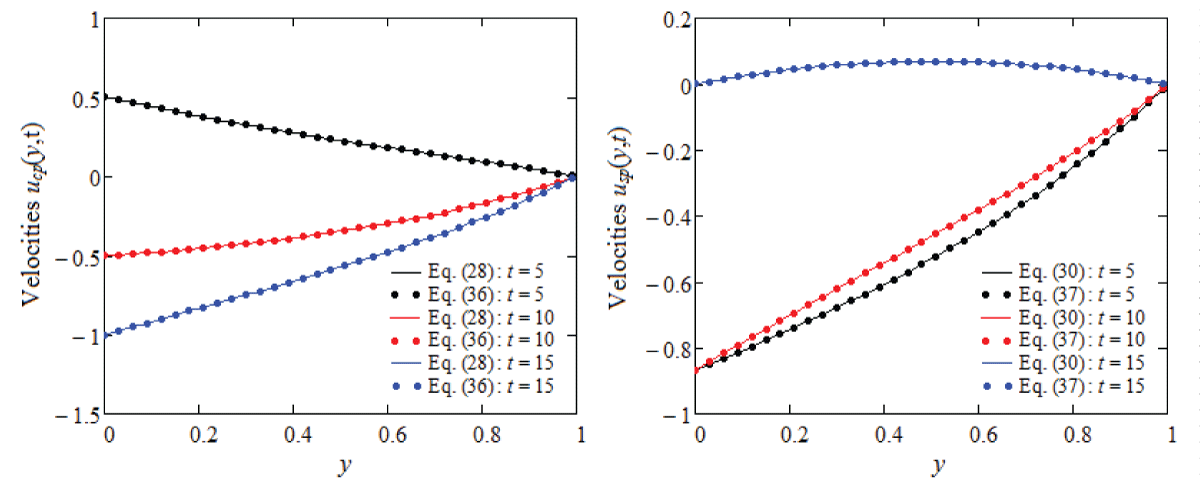
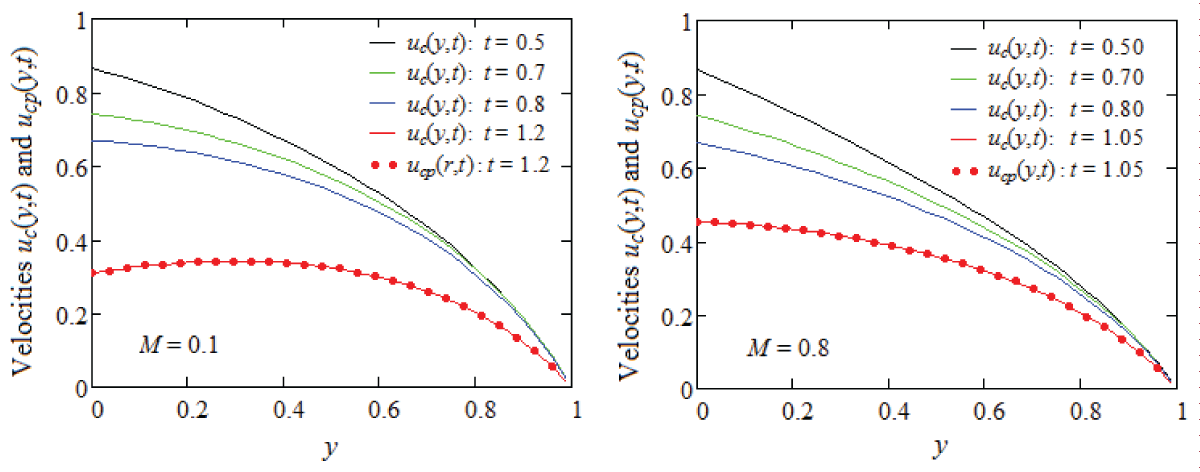
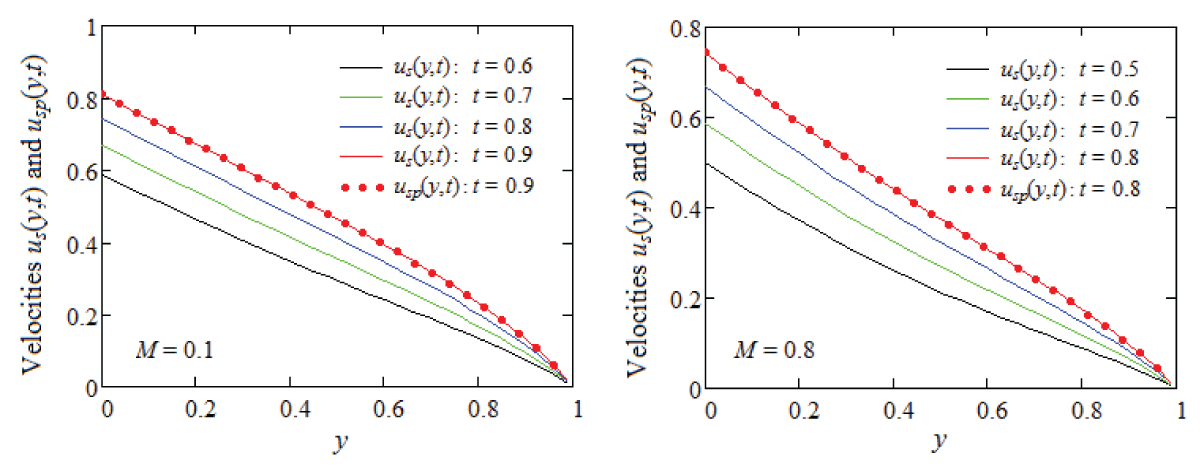
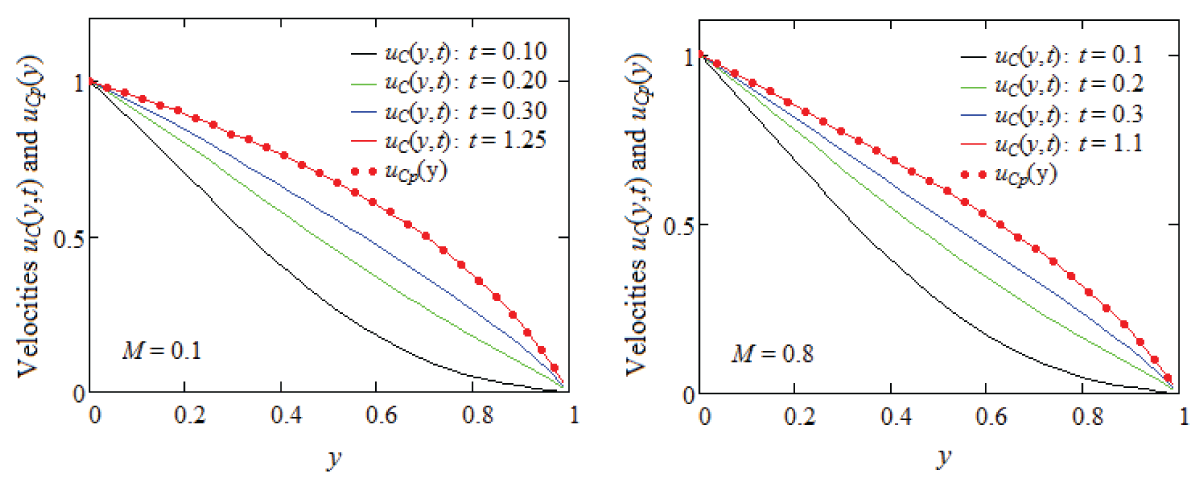
The analytical study examines the isothermal, unsteady flows of viscous incompressible fluids in a planar channel, when viscosity depends linearly on pressure, and a constant magnetic field is present. Exact expressions are derived for the dimensionless initial velocity field, the corresponding non-zero shear stress, and the problem is fully solved. To illustrate and highlight certain fluid behavior characteristics, modified Stokes’ problems are analyzed, and analytical expressions for the corresponding initial velocities are provided. For validation, the steady components are presented in two distinct forms, with their equivalence confirmed through graphical comparison. The effect of the magnetic field on the fluid behavior is explored and discussed visually. The results show that the fluid flows more slowly, and the steady-state is reached sooner when a magnetic field is applied.
2010 Mathematics Subject Classification: 76A05.

Figures

Flow geometry. Figure 1: Flow geometry....
Equivalence of the expressions of ucp(y,t) and usp(y,t) given by relations (28), (36) and (30), (37) for β = 0.5, M = 0.7, ω = Π/3 and three values of the time t. Figure 2: Equivalence of the expressions of ucp(y,t) and usp...
Convergence of starting velocity uc(y,t) from Eq. (27)1 to its steady state component ucp(y,t) for β = 0.2, ω = Π/3, two values of M and increasing values of time t. Figure 3: Convergence of starting velocity uc(y,t) from Eq. ...
Convergence of starting velocity us(y,t) from Eq. (27)2 to its steady state component usp(y,t) for β = 0.2, ω = Π/3, two values of M and increasing values of time t. Figure 4: Convergence of starting velocity us(y,t) from Eq. ...
Convergence of starting velocity uC(y,t) from Eq. (38)1 to its steady component uCp(y) for β = 0.2, ω = Π/3, two values of M and increasing values of time t. Figure 5: Convergence of starting velocity uC(y,t) from Eq. ...

References

    1. Stokes GG. On the theories of internal friction of fluids in motion, and of the equilibrium and motion of elastic solids. Trans Cambridge Philos Soc. 1945;8:287-305.
    2. Andrade C. Viscosity of fluids. Nature. 1930;125:309-310. doi: 10.1038/125309b0.
    3. Bridgman PW. The Physics of High Pressure. New York: The Macmillan Company; 1931.
    4. Cutler WG, McMicke RH, Webb W, Scheissler RW. Study of the compressions of several high molecular weight hydrocarbons. J Chem Phys. 1958;29:727-740. doi: 10.1063/1.1744583.
    5. Johnson KL, Cameron R. Shear behavior of elastohydrodynamic oil films at high rolling contact pressures. Proc Instn Mech Engrs. 1967;182(1):307-319. doi: 10.1243/PIME_PROC_1967_182_029_02.
    6. Johnson KL, Tevaarwerk JL. Shear behavior of elastohydrodynamic oil films. Proc R Soc Lond A. 1977;356:215-236. doi: 10.1098/rspa.1977.0129.
    7. Bair S, Winer WO. The high pressure high shear stress rheology of liquid lubricants. J Tribol. 1992;114(1):1-9. doi: 10.1115/1.2920862.
    8. Bendler JT, Fontanella JJ, Shlesinger MF. A new vogel-like law: ionic conductivity, dielectric relaxation, and viscosity near the glass transition. Phys Rev Lett. 2001 Nov 5;87(19):195503. doi: 10.1103/PhysRevLett.87.195503. Epub 2001 Oct 18. PMID: 11690421.
    9. Casalini R, Bair S. The inflection point in the pressure dependence of viscosity under high pressure: a comprehensive study of the temperature and pressure dependence of the viscosity of propylene carbonate. J Chem Phys. 2008 Feb 28;128(8):084511. doi: 10.1063/1.2834203. PMID: 18315065.
    10. Szeri AZ. Fluid Film Lubrication: Theory and Design. Cambridge: Cambridge University Press; 1998.
    11. Hron J, Malek J, Rajagopal KR. Simple flows of fluids with pressure dependent viscosities. Proc R Soc Lond A. 2001;457:1603-1622. doi: 10.1098/rspa.2000.0723.
    12. Rajagopal KR, Szeri AZ. On an inconsistency in the derivation of the equations of elastohydrodynamic lubrication. Proc R Soc Lond A. 2003;459:2771-2786. doi: 10.1098/rspa.2003.1145.
    13. Kannan K, Rajagopal KR. A model for the flow of rock glaciers. Int J Non-Linear Mech. 2013;48:59-64. doi: 10.1016/j.ijnonlinmec.2012.06.002.
    14. Rajagopal KR. Couette flows of fluids with pressure dependent viscosity. Int J Appl Mech Eng. 2004;9(3):573-585.
    15. Fetecau C, Bridges C. Analytical solutions for some unsteady flows of fluids with linear dependence of viscosity on the pressure. Inverse Probl Sci Eng. 2021;29(3):378-395. doi: 10.1080/17415977.2020.1791109.
    16. Rajagopal KR, Saccomandi G, Vergori L. Unsteady flows of fluids with pressure dependent viscosity. J Math Anal Appl. 2013;404:362-372. doi: 10.1016/j.jmaa.2013.03.025.
    17. Prusa V. Revisiting Stokes first and second problems for fluids with pressure-dependent viscosities. Int J Eng Sci. 2010;48:2054-2065. doi: 10.1016/j.ijengsci.2010.04.009.
    18. Fetecau C, Vieru D. Exact solutions for unsteady motion between parallel plates of some fluids with power-law dependence of viscosity on the pressure. Appl Eng Sci. 2020;1:100003. doi: 10.1016/j.apples.2020.100003.
    19. Vieru D, Fetecau C, Bridges C. Analytical solutions for a general mixed boundary value problem associated with motions of fluids with linear dependence of viscosity on the pressure. Int J Appl Mech Eng. 2020;25(3):181-197. doi: 10.2478/ijame-2020-0042.
    20. Yadav PK, Verma A. Analysis of the MHD flow of immiscible fluids with variable viscosity in an inclined channel. J Appl Tech Phys. 2023;64:618-627. doi: 10.1134/S0021894423040077.
    21. Verma AK. Numerical investigation of unsteady magnetohydrodynamic flow of a Newtonian fluid with variable viscosity in an inclined channel. Phys Fluids. 2025;37:013623. doi: 10.1063/5.0248969.
    22. Debnath L, Bhatta D. Integral Transforms and Their Applications. 2nd ed. Boca Raton: Chapman and Hall/CRC Press; 2007.
    23. Fetecau C, Morosanu C. Influence of magnetic field on Couette flow of fluids with linear dependence of viscosity on the pressure. Fundam J Math Math Sci. 2024;18(2):67-78.
Page Navigation
Authors Title Abstract Figures References Similar Articles View PDF
PUBLISH YOUR RESEARCH

We publish a wide range of article types in biology, medicine and engineering with no editorial biases.

Submit

See Manuscript Guidelines and APC

Explore the IgMin Subjects
Biology Medicine Engineering General Science

Which articles are now trending?

Click for top 10 Articles

Quick Links

Submit Manuscript
Subscribe to IgMin Research

Enter your e-mail address to stay informed about published articles, issue releases and latest updates on journal activities.

Research Articles
  1. Contribution to the Knowledge of Ground Beetles (Coleoptera: Carabidae) from Pakistan
  2. A Machine Learning-based Method for COVID-19 and Pneumonia Detection
  3. Technical & Economic Feasibility Study of Proposed Pump Storage Power Plants at Kuda Oya, Mul Oya, Gurugal Oya, and Dambagasthalawa
  4. Modeling of an Electric-fired Brick Oven, Directly Heated
  5. Enhancing Material Property Predictions through Optimized KNN Imputation and Deep Neural Network Modeling
  6. Benzo[4’,5’]imidazo[2’,1’:6,1]pyrido[2,3-d]pyrimidines: Past and Present
Read more

Advertisement

×

Why Publish with IgMin Research?

Submit Your Article